Determining the Original Voxel Densities

Introduction

In A-level Physics Remote Sensing, one particular concept that students were required to apply was the idea of using voxel to build up the x-ray image of a body. Some students were wondering the very arbitrary idea of performing certain procedures to arrive at the answer.

In this article, I am going to explain the reason why the series of steps work as described.

Procedure

Assuming four voxels with unknown densities,w, x, y and z, are investigated with an x-ray beam. When an x-ray beam is directed at the region, the detected intensity could be received at the other end. To simplify the idea, we will use addition of the densities of these different voxels, although a computer could always calculate the received intensities with the densities of voxels.

Step 1

By adding the densities on Direction 1, we receive the sum of densities along each row. In the upper row, we have w+x while the bottom row gives y+z. The upper red-highlighted voxels are added to the memory according to the same coloured locations.

Step 2

We then change the direction to Direction 2 and perform the same steps. However, we can see that the green and blue voxels produce only w and z respectively while the red voxels both produces x+y. These values are added to the momery locations as shown in Direction 2. .

Step 3

The same process is applied to Direction 3 and 4, and the values are added to the respective coloured locations as shown in .

Step 4

As your can observe, each memory location would be accumulated with a value of the sum of all voxels and its intrinsic value times 3. For example, in the top left voxel, it would take up a value of 4w + x + y + z. This is the reason why we need to subtract the background value, which is w + x + y + z.

After subtraction, each voxel would have a value of three times of its intrinsic value(3w for the top left voxel). This is the reason why we need to divide the values by 3 to get the original intrinsic values of each voxel.

Example

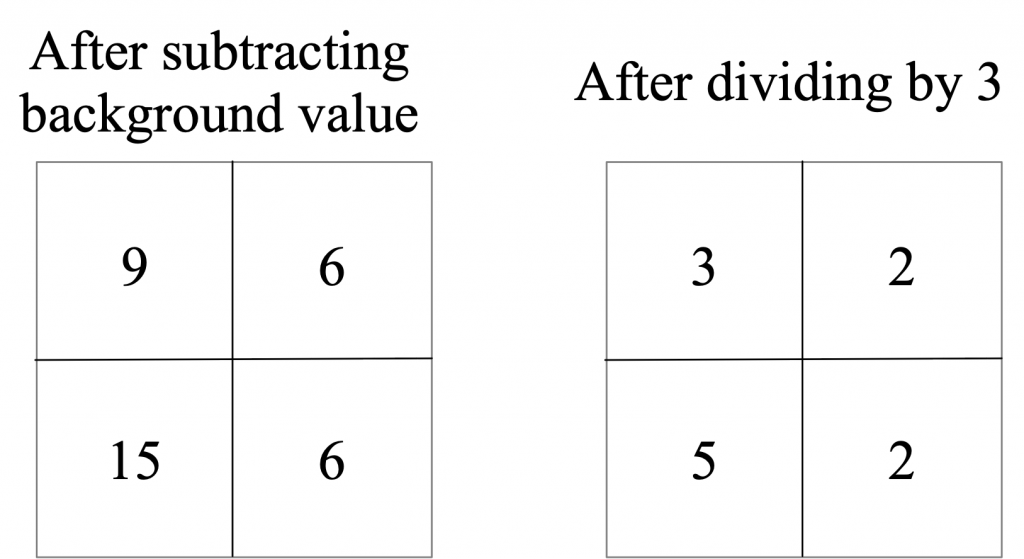
Let us use one example with values.

Subtract each voxel with the background value of 12.

Divide by three throughout, we get back the original voxel values in their corresponding locations.

Conclusion

The seemingly random series of steps confuses many students when I was teaching this concept. I hope that by using general algebraic notation, you could see the reason why the values are added, subtracted and divided as described by many A-level textbooks.